It’s been 24 hours since my last post, and I’ve come to see that part of the problem is that people don’t understand what the word “exponential” means.

The word exponential has been floating around a lot lately as concern about COVID-19 grows and spreads around the world. It’s perhaps the most important word in understanding all of this, and since it’s been a while since most of us took a math class, I thought this would be a good time to review the concept and how it relates to the current pandemic.

So first. What does exponential growth mean? It means that the growth is increasing at a faster and faster rate. When it comes to the spread of a virus, an exponential growth rate means that as we go from one day to the next, the number of cases multiplies by some constant number that’s greater than one. I’ll also explain how physical distance plays into the equation and why that will save the life of your grandmother (and mine).

Check out this video by 3Blue1Brown for a better explanation of the math than the abbreviated version I’m going to put here:

The spread of viruses is a perfect example of something that grows exponentially because the number of new cases each day is based on the number of cases yesterday.

If the number of cases on a day is “N” and each person with the virus is exposed to “E” people in a day, and each interaction has the probability “P” of resulting in a new infection, then the number of new cases is (number of cases) x (how many people) x (probability of infection). Or,

Change in N = N*e*P

See how N is on both sides of that equation?

That’s what makes things speed up quickly. Because if N gets big, the rate of change gets big - and it keeps picking up speed as N gets bigger.

Think about it this way, as explained in an (unrelated) article from The College Investor. Would you rather have $1,000 now or a penny that doubles every day for the next thirty days? At first glance, you might say $1,000. How can a penny turn into more than that by doubling in 30 days?

In the first six days, the penny goes from $0.01 to $0.32. Not doing so well. But by the time you reach day fifteen, that’s $163.84. A far way off from the $1,000 I turned down at the beginning, right?

Keep going.

By day twenty - just five days later, we’re now at $5,242.88.

By the end of the month? You have $5,368,709.12.

Now take that penny and imagine it’s people. One person with a virus isn’t a big deal (except to that person). But let that one start doubling, and let it go unchecked, and that’s how you end up with 181 million cases across 161 countries (as of this writing on the afternoon of March 16).

Going back to the above video to look at COVID-19 cases outside mainland China, we can see that it took 20 days to go from 100 cases to 1,000 cases - but just 13 days to go from 1,000 to 10,000 cases. Currently, the number of cases appears to multiply by 10 every 16 days on average.

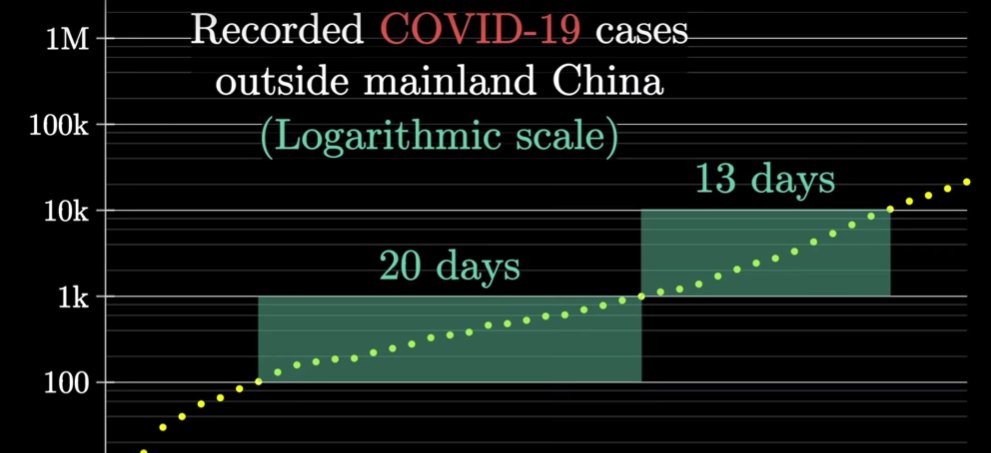
This segment of the video helps explain a tricky misconception.

If you see a country with 65 cases and one with 6,500 cases, it’s easy to think that one is doing 100x better than the other. But if you’re actually in a situation where the number of cases multiplies by ten every sixteen days, you can see that the second country is a month behind the first. If that trend continued, you’ll hit a million cases in thirty days, ten million in 47 days, 100 million in 64 days, and one billion in 81 days.

The good news is that exponential growth can’t continue forever. But as they say in the video, “The crucial question is when.” The SARS outbreak in 2002 ended with about eight thousand cases - while the Spanish Flu of 1918 infected 27% of the world.

In the worst possible scenario, the exponential growth would continue until it reached the full population before declining. The goal is to end the exponential curve as quickly as possible.

To understand how contact spreads a virus, and how limiting contact can curb that spread, check out this interactive blog post from the Washington Post. In it, you’ll see how a virus spreads from person to person in various scenarios, including attempted mandatory isolation.

Part of how other countries have been successful at decreasing their growth curve lies in government regulations. When the government bans social gatherings, requires businesses to shut down, closes schools, etc. then people are more likely to follow recommendations to stay home.

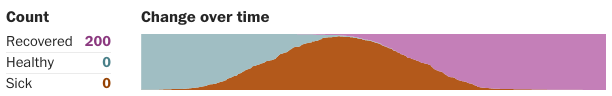
As seen in the Washington Post simulation referenced above, you can see that if ¾ of the population stays home and ¼ moves around freely (for example, healthcare workers and other essential services), the curve changes from this, where everyone moves around freely:

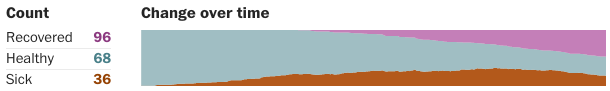
To this:

(Both examples don’t take into account death, which is an unfortunate certainty with the spread of this virus).

The first scenario shows the worst-case example of exponential growth, in which the number of cases rapidly increases in a short amount of time until the full population has become infected. Our healthcare systems cannot support that level of demand if this were to happen in reality, and millions of people would die due to lack of care.

The second scenario, with limited mobility and physical distancing, results in lower strain on the healthcare system as people get sick at a slower rate AND a large portion of the population that never gets sick. This is the ideal scenario to promote the survival of the maximum number of people.

There’s no question that a lot of people are going to get sick and many will die from this. What we’re trying to do is prevent MORE people from dying purely because we don’t have the ICU beds and equipment to treat them. The only way to do this is to “flatten the curve” by limiting contact, so that fewer people get sick at once and our healthcare system can keep up.

In sum, I think 3Blue1Brown says it best:

“If people are sufficiently worried, there’s a lot less to worry about. If no one is worried, that’s when you should worry.”

ABOUT THE AUTHOR:

Dr. Greg Jacobson is the CEO and co-founder of KaiNexus. He's also an ER doctor of 20 years and is married to a double-boarded doctor with more experience. He realizes that his family has a unique perspective, and therefore, he has a duty and obligation to educate and influence as many people as possible about preventing the spread of COVID-19.